Question

Given the position of the particle, what the position(s) of the particle when it’s at rest

Answer 1

The position function of a particle is given by:

`X\mleft(t\mright)=(2)/(3)t^3-(9)/(2)t^2-18t`

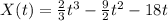
The velocity function is the derivative of the position:

`\begin{gathered} V(t)=(2)/(3)(3t^2)-(9)/(2)(2t)-18 \\ \text{Simplifying:} \\ V(t)=2t^2-9t-18 \end{gathered}`

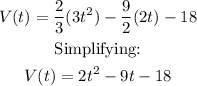
The particle will be at rest when the velocity is 0, thus we solve the equation:

`2t^2-9t-18=0`

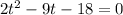
The coefficients of this equation are: a = 2, b = -9, c = -18

Solve by using the formula:

`t=\frac{-b\pm\sqrt[]{b^2-4ac}}{2a}`

Substituting:

`\begin{gathered} t=\frac{9\pm\sqrt[]{81-4(2)(-18)}}{2(2)} \\ t=\frac{9\pm\sqrt[]{81+144}}{4} \\ t=\frac{9\pm\sqrt[]{225}}{4} \\ t=(9\pm15)/(4) \end{gathered}`

We have two possible answers:

`\begin{gathered} t=(9+15)/(4)=6 \\ t=(9-15)/(4)=-(3)/(2) \end{gathered}`

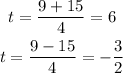
We only accept the positive answer because the time cannot be negative.

Now calculate the position for t = 6: