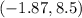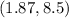Question

Find the points on the curve y=x2+5 closest to the point (0,9). Round to the nearest two decimal places. Write the point with the smaller x value first.

Answer 1

The equation for the distance between any point and the point (0, 9) is:

`d=√(x^2+(y-9)^2)`

The curve given in the problem is:

`y=x^2+5`

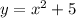
If we solve for 'x²', we can substitute in the distance equation and obtain a function for the distance of any point in the curve to (0, 9):

`x^2=y-5`

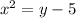
And substitute:

`d(y)=√((y-5)+(x-9)^2)`

SImplify:

`d(y)=√(y^2-17y+76)`

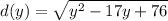
And now, we need to calculate the first and second derivatives:

`d^(\prime)(x)=(2y-17)/(2√(y^2-17y+76))`
`d^(\prime)^(\prime)(y)=\frac{15}{4(y^2-17y+76)^{(3)/(2)}}`

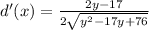
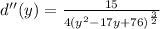
Then, find the critical points of d(y). Since this function is a quotient, it will be equal to 0 when the numerator is equal to 0:

`\begin{gathered} 2y-17=0 \\ . \\ y=(17)/(2) \end{gathered}`

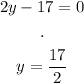
Now, evaluate this value into the second derivative:

`d^(\prime)^(\prime)((17)/(2))=\frac{15}{4(((17)/(2))^2-17\cdot(17)/(2)+76)^{(3)/(2)}}\approx0.516546`

Since is a positive value, the function d(y) has a minimum in y = 17/2

Next, we need to find the values of x. We use the equation of the curve:

`\begin{gathered} (17)/(2)=x^2+5 \\ . \\ x^2=(17)/(2)-5 \\ . \\ x=\pm\sqrt{(7)/(2)}=\pm(√(14))/(2) \end{gathered}`

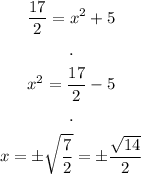
Thus, the point on the curve closest to (0, 9), rounded to two decimals, are:

`(-1.87,8.5)`
`(1.87,8.5)`