Question

A company will need 1.8 million 5 years from now to replace some equipment. Currently, the firm has some extra cash and would like to establish a savings account for this purpose. The account pays 5.25 percent interest, compounded annually. How much money must the company deposit today to fully fund the equipment purchase?

Answer 1

Given:

a.) A company will need 1.8 million 5 years from now to replace some equipment.

b.) The account pays 5.25 percent interest, compounded annually.

We will be applying the Compounded Interest Formula:

`\text{ A = P\lparen1 + }(r)/(n))^(nt)`

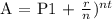
Where,

A=final amount

P=initial principal balance/money to initially deposit

r=interest rate (decimal)

n=number of times interest applied per time period

t=number of time periods elapsed (in years)

In this scenario, we are asked what is the amount of principal balance/initial deposit to make to get 1.8 million in 5 years.

Annually = n = 1

We get,

`\text{ 1,800,000 = P\lparen1 + }((5.25)/(100))/(1))^((1)(5))`
`1,800,000\text{ = P \lparen1 + 0.0525\rparen}^5\text{ = P \lparen1.0525\rparen}^5`
`\text{ P = }(1,800,000)/((1.0525)^5)`
`\text{ P = 1,393,676.5175887556 }\approx\text{ 1,393,676.52}`

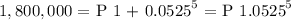
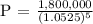
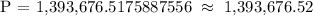
Therefore, the answer is 1,393,676.52