Question

Flying against the wind, a jet travels 6320 miles in 8 hours. Flying with the wind, the same jet travels 5950 miles in 5 hours. What is the rate of the jet in stillair and what is the rate of the wind?Rate of the jet in still air:Rate of the wind:

Answer 1

Given,

A jet travels 6320 miles in 8 hours.

Flying with the wind, the same jet travels 5950 miles in 5 hours.

To find: The rate of the jet in still air, and the rate of the wind.

Solution:

The jet's velocity against the wind is

`(6320)/(8)=790\text{ miles per hour}`

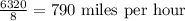
The jet's velocity with wind is

`(5950)/(5)=1190\text{ miles per hour}`

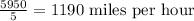
Let the velocity of the jet in still air be x miles per hour and velocity of wind be y miles per hour.

As such its velocity against wind is x-y and with wind is x+y and therefore

`\begin{gathered} x-y=790.......(1) \\ x+y=1190.......(2) \end{gathered}`

Solve both equations (1) and (2)

`\begin{gathered} 2x=1980 \\ x=(1980)/(2) \\ x=990 \end{gathered}`

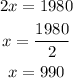
And

`\begin{gathered} y=1190-990 \\ y=200 \end{gathered}`

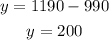
Hence, the velocity of the jet in still air is 990 miles per hour and the velocity of wind is 200 miles per hour.