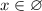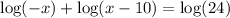
start by applying the log properties on the left side
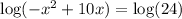
remember that when we talk about the logs they are defined for values greater than 0, determine the range in which will be defined
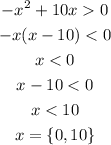
knowing that the values in order for the log to be true must be between 0 and 10, apply base 10 on both sides to cancel the log
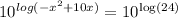
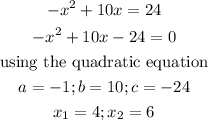
after having the values of the quadratic replace them on the equation
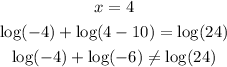

after seeing that with both solutions is false the statement, we can conclude that there are no solutions for x.