Answer:
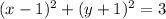
Explanation:
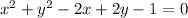
Standard form of the equation is

To get standard form we apply completing the square method

Take coefficient of x and y . Divide it by 2 and then square it
 and 1^2=1
and 1^2=1
Add and subtract 1
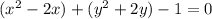

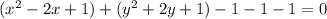
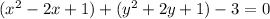
Now write the parenthesis in square form
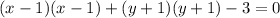
 , add 3 on both sides
, add 3 on both sides
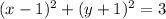 is the standard form
is the standard form