Question

A milkshake vendor sells shakes in three different flavors: see photo

Answer 1

Let the price of mango, strawberry and chocolate flavors be x, y, and z respectively.

Thus,

`\begin{gathered} mango\Rightarrow x \\ strwberry\Rightarrow y \\ chocolate\Rightarrow z \end{gathered}`

From the table below:

Given that the total sales in shift 1 is $419, $649 in shift 2, and $96 in shift 3, this implies that

`\begin{gathered} 16x+13y+29z=419\text{ ----- equation 1} \\ 26x+19y+45z\text{ = 649 ----- equation 2} \\ 6x+11y+0z\text{ = 96 ------ equation 3} \end{gathered}`

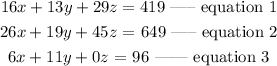
From the above system of equations, we can resolve into matrices of the form:

`A\cdot X=B`

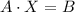
This gives:

`\begin{bmatrix}{16} & {13} & {29} \\ {26} & {19} & {45} \\ {6} & {11} & {0}\end{bmatrix}*\begin{bmatrix}{x} & {} & {} \\ {y} & {} & {} \\ {z} & {} & {}\end{bmatrix}=\begin{bmatrix}{419} & {} & {} \\ {649} & {} & {} \\ {96} & {} & {}\end{bmatrix}`

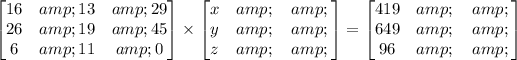
Where:

`\begin{gathered} A=\begin{bmatrix}{16} & {13} & {29} \\ {26} & {19} & {45} \\ {6} & {11} & {0}\end{bmatrix} \\ X=\begin{bmatrix}{x} & {} & {} \\ {y} & {} & {} \\ {z} & {} & {}\end{bmatrix} \\ B=\begin{bmatrix}{419} & {} & {} \\ {649} & {} & {} \\ {96} & {} & {}\end{bmatrix} \end{gathered}`

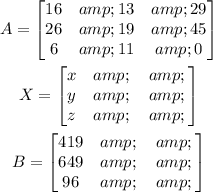
To solve for the unknowns, we use the Cramer's rule expressed s

`\begin{gathered} x=(D_x)/(D) \\ y=(D_y)/(D) \\ z=(D_z)/(D) \\ where\text{ D is the determinant of the matrix A} \end{gathered}`

To evaluate the price of one chocolate, which is z, we have

`\begin{gathered} z=(D_z)/(D)=\frac{det\begin{bmatrix}{16} & {13} & {419} \\ {26} & {19} & {649} \\ {6} & {11} & {96}\end{bmatrix}}{det\text{ A}} \\ =\frac{16*\det\begin{pmatrix}19 & 649 \\ 11 & 96\end{pmatrix}-13*\det\begin{pmatrix}26 & 649 \\ 6 & 96\end{pmatrix}+419*\det\begin{pmatrix}26 & 19 \\ 6 & 11\end{pmatrix}}{578} \\ =(16(-5315)-13(-1398)+419(172))/(578) \\ =(5202)/(578) \\ \Rightarrow z=9 \end{gathered}`

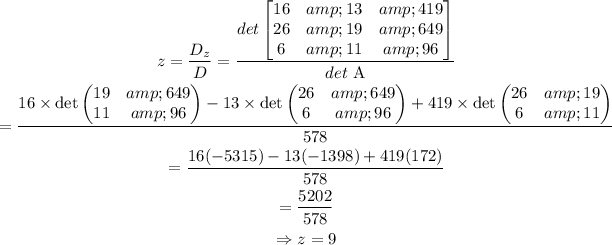
Hence, the price of one chocolate is

`\$9`

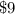
The correct option is D