Answer: It can be expressed as

Explanation:
Since we have given that 0.44444.......
We need geometric series that represents as a fraction.
so, it can be written as
0.4+0.04+0.004+0.0004...............
But as we are required to write it as a fraction , So, it becomes,
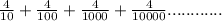
and it is a geometric series.
Because it has first term = a =
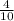
and common ratio = r =
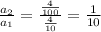
Hence, it can be expressed as
