Question

WHAT IS 1/3 TO THE TENTH POWER IN FRACTION FORM?

AND WHAT IS (1/3)^10 x 9^4
`(( (1)/(3)) ^(10)) 9^4`

1/3 to the tenth power times 9 to the 4th power

Answer 1

1/3 ^ 10 = 1/3 * itself ten times.
1/59049 x 9 ^ 4
1/59049 x 6561 = 6561/59049
1/9

Answer 2

Well, 1/3 to the 10th power. Since the numerator is 1, just ignore that.

Find the tenth power of 3.
3 x 3 x 3 x 3 x 3 x 3 x 3 x 3 x 3 x 3 = 59049
So, 1/3 to the 10th power is equal to 1/59049.

`(1)/(3) ^(10) = (1)/(59049)`

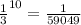
Okay, for the second equation we know that 1/3 to the tenth power is already 1/59049.

Now find the 4th power for 9.

9 x 9 x 9 x 9 = 6561

`9*9*9*9=6561`

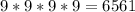
Convert 6561 into a fraction, which is 6561/1.


Now multiply.

1/59049 x 6561/1 = 6561/59049

That fraction reduces into 1/9.

Answer #1: 1/59049
Answer #2: 1/9