Question

Find f^-1(x)= 2x/ 2+3x make sure its 1-1, if so find its inverse

Answer 1

The given function is:

`f(x)=(2x)/(2+3x)`

Graph the function as shown below:

As seen from the horizontal line test, the lines cut the function at only 1 point.

Hence the function is 1-1 and therefore invertible.

`f(x)=y=(2x)/(2+3x)`

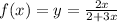
Solve for x to get:

`\begin{gathered} 2y+3xy=2x \\ 2y=2x-3xy \\ 2y=x(2-3y) \\ x=(2y)/(2-3y) \end{gathered}`
`\text{ Since y=f(x),x=f}^(-1)(y)`

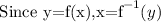
Therefore replace y by x to get the required inverse function shown below:

`\begin{gathered} x=f^(-1)(y)=(2y)/(2-3y) \\ f^(-1)(x)=(2x)/(2-3x) \end{gathered}`

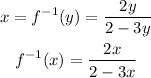
The inverse function is shown above.