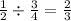Question

Select whether the quotient for each fraction division expression is less than one or greater than/ equal to one.​

Answer 1

(Always be happy) ok.56

Answer 2

`\( (1)/(2) / (3)/(4) = (2)/(3) \)`, a quotient less than one. Other expressions yield quotients greater than one.

Certainly! Let's analyze the given fraction division expressions step by step.

1.
`\( (1)/(2) / (3)/(4) \)`

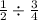
To divide fractions, we multiply the first fraction by the reciprocal of the second. The reciprocal of
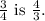`\( (3)/(4) \) is \( (4)/(3) \).` So, the calculation is:

`\( (1)/(2) * (4)/(3) = (1 * 4)/(2 * 3) = (4)/(6) \)`

Now, simplify the fraction:

`\( (4)/(6) = (2)/(3) \)`

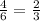
The quotient
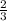`\( (2)/(3) \)` is less than one.

2.
`\( (5)/(6) / (2)/(3) \)`

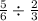
Again, we multiply the first fraction by the reciprocal of the second
`(\( (3)/(2) \)):`

`\( (5)/(6) * (3)/(2) = (5 * 3)/(6 * 2) = (15)/(12) \)`

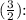
Simplify the fraction:

`\( (15)/(12) = (5)/(4) \)`

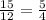
The quotient
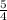`\( (5)/(4) \)` is greater than one.

3.
`\( (4)/(5) / (1)/(2) \)`

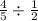
Multiply the first fraction by the reciprocal of the second
`(\( (2)/(1) \)):`

`\( (4)/(5) * (2)/(1)` =
`(4 * 2)/(5 * 1)` =
`(8)/(5) \)`

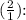
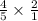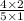The quotient
`\( (8)/(5) \)` is greater than one.

In conclusion, only the first expression
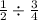`\( (1)/(2) / (3)/(4) \)` has a quotient less than one, while the other two expressions have quotients greater than one.