Question

Show that the points (0.-1).(2, 1).(0.3) and (-2, 1) are the vertices of a square ABCD.

O.math​

Answer 1

The diagram is below

• A = (0,-1)
• B = (2,1)
• C = (0,3)
• D = (-2,1)

Let's find the distance from A to B using the distance formula.

`d = √((x_1 - x_2)^2 + (y_1 - y_2)^2)\\\\d = √((0-2)^2 + (-1-1)^2)\\\\d = √((-2)^2 + (-2)^2)\\\\d = √(4 + 4)\\\\d = √(8)\\\\d \approx 2.828\\\\`

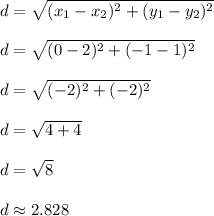
The distance from A to B is exactly
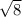`√(8)` units or approximately 2.828 units. This is the length of side AB.

Through very similar steps, you should find that the other side lengths of BC, CD and AD are all the same.

Since AB = BC = CD = AD, this means we have a rhombus. Recall a rhombus is any quadrilateral with all four sides the same length.

-----------------------------

Let's find the slope of line AB

`m = (y_(2) - y_(1))/(x_(2) - x_(1))\\\\m = (1 - (-1))/(2 - 0)\\\\m = (1 + 1)/(2 - 0)\\\\m = (2)/(2)\\\\m = 1\\\\`

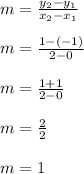
Line AB has a slope of 1.

Through similar steps, you should find the slope of line BC is -1.

Rule: Whenever two slopes multiply to -1, then their corresponding lines are perpendicular.

Based on that rule, lines AB and BC are perpendicular. This means they meet up at a right angle (aka 90 degree angle).

You should find that line CD has a slope of 1, so BC and CD are perpendicular. Lastly, line AD has a slope of -1. So all the slopes mentioned are either +1 or -1. The slopes alternate as you move around the figure. This all shows that any two adjacent sides meet at a 90 degree angle.

Therefore, this figure is a rectangle. Any rectangle has all four angles of 90 degrees.

-----------------------------

The first section showed that we have a rhombus. The second section afterward showed that we also have a rectangle. The combination of a rhombus and a rectangle at the same time leads to a square.

Therefore, quadrilateral ABCD is a square.