Question

a phone company offers two monthly charge plans. in plan A there is no monthly fee, but the customer pays 6 cents per minute of use. in plan B the customer pays a monthly fee of $ 9.40 and then an additional 4 cents per minute of use.for what amount of monthly phone use will plan A cost at least as much as plan B?use m for the number of minutes of phone use in a month, and solve your inequality for m?

Answer 1

470 minutes

Step-by-step explanation:

Let the number of minutes of phone used in a month = m

Plan A

The customer pays 6 cents per minute of use.

Therefore, the total cost for plan A = 0.06m

Plan B

The customer pays a monthly fee of $9.40 and then an additional 4 cents per minute of use.

Therefore, the total cost for plan B = 9.40+0.04m

If plan A costs at least as much as plan B, then we have:

`0.06m\ge9.40+0.04m`

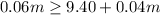
We then solve for m.

`\begin{gathered} 0.06m-0.04m\ge9.40 \\ 0.02m\ge9.40 \\ m\ge(9.40)/(0.02) \\ m\ge470 \end{gathered}`

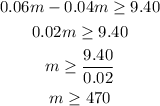
When you use the phone for at least 470 minutes, the amount of monthly phone use for plan A will cost at least as much as plan B.