Question

Please solve, fill in the blank for each (a) through (d)

Answer 1

We are given the following function:

`f(x)=(x)/(x-8),g(x)=-(1)/(x)`

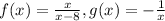
Part A. We are asked to determine the following:

`f\circ g`

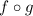
This means the composition of the function "f" and "g". To do that we will use the following equivalence:

`(f\circ g)(x)=f(g(x))`

This means that we will substitute the value of "x" from function "f" by the function "g", like this:

`f(g(x))=(-(1)/(x))/(-(1)/(x)-8)`

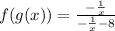
Now, we simplify the fraction:

`f(g(x))=(1)/(1+8x)`

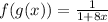
To determine the domain we must set the denominator to zero to determine the values of "x" for which the composition of the functions is undetermined:

`1+8x=0`

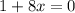
Now, we solve for "x". First, we subtract 1 from both sides:

`8x=-1`

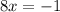
Now, we divide both sides by 8:

`x=-(1)/(8)`

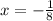
Therefore, the composition is undetermined at the point "x = -1/8". Therefore, the domain is:

`D={}\lbrace x\parallel x<-(1)/(8),x>-(1)/(8)\rbrace`

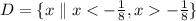
Part B. we are asked to determine the following:

`g\circ f`

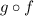
This is equivalent to:

`(g\circ f)(x)=g(f(x))`

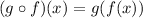
This means that we will substitute the value of "x" from function "g" for the value of function "f", like this:

`g(f(x))=-(1)/((x)/(x-8))`

Now, we simplify the expression:

`g(f(x))=-(x-8)/(x)`

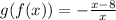
To determine the domain we set the denominator to zero:

`x=0`

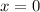
Therefore, the function is undetermined at "x = 0". This means that the domain is the values of "x" that do not include the value of "x = 0":

`D=\lbrace x\parallel x>0,x<0\rbrace`

Part C. We are asked to determine:

`f\circ f`

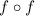
This is equivalent to:

`(f\circ f)(x)=f(f(x))`

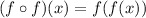
Now, we substitute the value of "x":

`f(f(x))=((x)/(x-8))/((x)/(x-8)-8)`

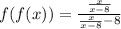
Now, we multiply the numerator and denominator by "x - 8":

`f(f(x))=(x)/(x-8(x-8))`

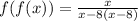
Applying the distributive property and adding like terms we get:

`f(f(x))=(x)/(x-8x+64)=(x)/(-7x+64)`

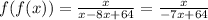
Now, we set the denominator to zero to determine the domain:

`-7x+64=0`

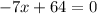
Now, we subtract 64 from both sides:

`-7x=-64`

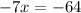
Now, we divide both sides by -7:

`x=(64)/(7)`

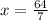
Therefore, the domain does not include the value of "x = 64/7". Therefore, the domain is:

`D=\lbrace x\parallel x<(64)/(7),x>(64)/(7)\text{ \textbraceright}`

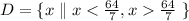
Part D. We are asked to determine:

`(g\circ g)(x)=g(g(x))`

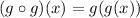
Substituting the value of "g(x)" in "g(x)" we get:

`g(g(x))=-(1)/(-(1)/(x))`

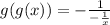
Simplifying we get:

`g(g(x))=x`

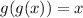
To determine the domain we need to have into account that the function is a polynomial and therefore, is not undetermined at any point. This means that the domain is all the real numbers.