Question

A ship is sailing 7 miles per hour with a direction angle of 25 degrees. On deck, the captain fires a cannonball that flies 100 miles per hour with a direction angle of 280 degrees.What is the direction angle of the cannonballs resultant vector?

Answer 1

283.9°

Explanation:

We must calculate the components of each vector, add the corresponding components to obtain the components of the resulting vector, like this:

Knowing the components of the resulting vector, calculate the angle, as follows:

`\begin{gathered} \tan\theta=(y)/(x) \\ \\ \tan\theta=(-95.52)/(23.7) \\ \\ \theta=\:\tan^(-1)\left((-95.52)/(23.7)\right) \\ \\ \theta=-76.06541\degree \\ \\ \text{ In the Cartesian plane at an angle it will be:} \\ \\ \theta=360\degree-76.06541\degree=283.93459\degree \\ \\ \theta=283.9\degree \end{gathered}`

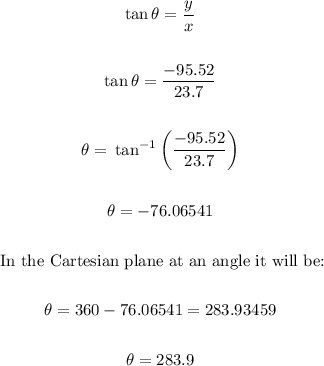
The angle of the resulting vector is 283.9°