Question

Which of the following is not a valid probability distribution for a discrete random variable? Check all that apply.

A. 1/5,1/10 ,1/10 ,1/10 ,1/5 ,1/10 ,1/10 , 1/10

B. 1/3,1/4 ,1/5 ,1/6

C. 1/2,1/4 ,1/8 ,1/16 ,1/32 ,1/64 ,1/128 ,1/128

D. -1/2, -1/3, -1/4, -1/5, 137/60

E. 1/6, 1/6, 1/6, 1/6, 1/6, 1/6

Answer 1

Option B and D are correct.

Explanation:

We know that Sum of the probability distribution of a discrete random variable is equal to 1.

using this result we check which is not valid probability distribution.

Option A).

Sum of Probability Distribution

`=(1)/(5)+(1)/(10)+(1)/(10)+(1)/(10)+(1)/(5)+(1)/(10)+(1)/(10)+(1)/(10)=(2+1+1+1+2+1+1+1)/(10)=(10)/(10)=1`

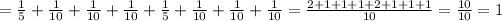
So, This is valid Probability distribution.

Option B).

Sum of Probability Distribution

`=(1)/(3)+(1)/(4)+(1)/(5)+(1)/(6)=(20+15+12+10+)/(60)=(57)/(10)\\eq1`

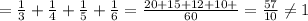
So, This is not a valid Probability distribution.

Option C).

Sum of Probability Distribution

`=(1)/(2)+(1)/(4)+(1)/(8)+(1)/(16)+(1)/(32)+(1)/(64)+(1)/(128)+(1)/(128)=(64+32+16+8+4+2+1+1)/(128)=(128)/(128)=1`

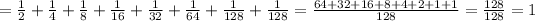
So, This is valid Probability distribution.

Option D).

Given Probability Distribution has negative probability which is not possible.

So, This is not a valid Probability distribution.

Option E).

Sum of Probability Distribution

`=(1)/(6)+(1)/(6)+(1)/(6)+(1)/(6)+(1)/(6)+(1)/(6)=(1+1+1+1+1+1)/(6)=(6)/(6)=1`

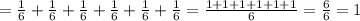
So, This is valid Probability distribution.

Therefore, Option B and D are correct.