Given:
There is a diagram given in the question
Required:
We need to find the equation of parabola and focus
Step-by-step explanation:
As we can see that vertex point of parabola is origin
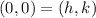
the general equation of parabola is
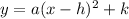
now substitute the values
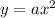
now by diagram there is a point

we use this point to find the a
Plug the point in equation
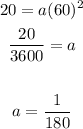
Now the equation of parbola is
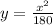
now the coordinate of focus is
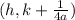
substitute all the values
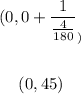
Final answer:
Coordinate of focus is
