Question

Bobby's Christmas list had 3 more than twice as many items on it than Johnny's list. Jhonny asked for 8 fewer items than bobby. How many items were on Johnny's (x) and Bobby's (y) Christmas lists

Answer 1

because jonny asked for 8 fewer items than bobby and bobby's list had 3 more than twice that of johny, the "twice" is 5. Two possible answers for this problem could be 13 and 5 since they both satisfy the requirement. if you can't logically deduct a function for it, look at the problem closely, then test and guess! Have fun with math!

Answer 2

Bobby made the Christmas list with 13 items, and Johnny with only 5 items.

Explanation:

"Bobby's Christmas list hat 3 more than twice as many items than Johnny's". This statement is represented by:

`B=2J+3`; where
`B` is Bobby, and
`J` is Johnny.

Then, the problem states, "Johnny asked for 8 fewer items than bobby", it's represented by:

`J=B-8`

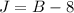
Now, to find the value of each variable, we solve this system of equations by substitution:

`B=2J+3\\B=2(B-8)+3\\B=2B-16+3\\13=2B-B\\B=13`

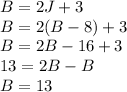
Then, we replace this into second equation to find
`J`:

`J=B-8=13-8=5`

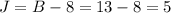
Therefore, Bobby made the Christmas list with 13 items, and Johnny with only 5 items.