Question

A student inscribes a triangle within a semicircle. What is the measure of ∠XYZ?

a. 90°
b. 60°
c. 45°
d. 120°

Answer 1

we know that

The inscribed angle measures half that of the arc that comprises

In this problem

∠XYZ------> is the inscribed angle

∠XYZ=
`(1)/(2)[arc\ XZ]`

`arc\ XZ=180\°` ------> because is a diameter of the circle

substitute

∠XYZ=
`(1)/(2)[180\°]=90\°`

therefore

the answer is the option A

`90\°`

Answer 2

Answer: a. 90°

Explanation:

We know that the in a circle, the measure of an inscribed angle is half the measure of the central angle with the same intercepted arc.

In the problem∠XYZ is the inscribed angle

∠XYZ=
`(1)/(2)\ arc(XZ)`

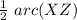
⇒ ∠XYZ=
`(1)/(2)\angle{XZ}`

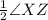
Since XZ is a diameter of the circle which is a line segment, thus ∠XZ=180°

∴ ∠XYZ=
`(1)/(2)180^(\circ)=90^(\circ)`

∴ ∠XYZ=
`90^(\circ)`

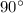
Therefore, a. 90° is the measure of ∠XYZ.