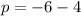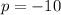Question

the axis of symmetry of a quadratic equation is x = –3. if one of the zeroes of the equation is 4, what is the other zero?

Answer 1

-10

Explanation:

i just took the test

Answer 2

ANSWER

The other zero is


`x = - 10`

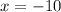
EXPLANATION

The axis of symmetry serves as the midpoint of the two zeroes.


We were given that the axis of symmetry of the quadratic equation is

`x = - 3`


We were also given that, one of the zeroes of the quadratic equation is

`x = 4`


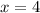
Let the other zero of the quadratic equation be

`x = p`

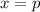
,then, we apply the midpoint formula to find the value of

`p`

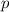
Since it is the x-values of the intercepts that gives the solution, we use only the x-value part of the midpoint formula which is given by,





`(x_1 + x_2)/(2) = axis \: of \: symmetry`

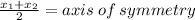
We substitute to obtain,


`(p + 4)/(2) = - 3`



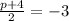
We multiply through by 2 to get,


`p + 4 = - 6`



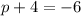
This implies that,


`p = - 6 - 4`


`p = - 10`