Question

. Segment JK has coordinates J(3, –6) and K(–3, 2). When segment JK is dilated with a scale factor –4, what are the coordinates of J' and K'?

A ) J'(–1, –10) and K'(–7, –2)
B ) J'(7, –2) and K'(1, 6)
C ) J'(–3/4, 3/2) and K'(3/4, –1/2)
D ) J'(–12, 24) and K'(12, –8)

Answer 1

Answer: D) J'(–12, 24) and K'(12, –8)

Explanation:

A dilation is a type of transformation that changes that size of a figure which is known as the pre-image to produce a new figure known as the image.

The rule to dilate a figure is to multiply the scale factor to the coordinates of the pre-image to get the coordinates of the image .

Therefore, If scale factor =-4

Then, The coordinates of J' =
`J'(x,y)=J'(-4(3),-4(-6))=J'(-12,-24)`

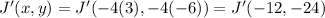
The coordinates of K' =
`K'(x,y)=K'(-4(-3),-4(2))=K'(12,-8)`

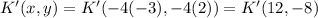
The coordinates of J' and K' are J'(–12, 24) and K'(12, –8).

Hence, D is the right answer.

Answer 2

the answer is D ) J'(–12, 24) and K'(12, –8)
proof
(–12, 24)= -4x(3, –6) and (12, –8)= -4x(–3, 2)