Answer: The shortest distance between the tip of the cone and its rim is 51.15 cm.
Explanation:
Since we have given that
Angle between the sloping side = 77°
Angle will get divided and the angle will be as
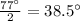
In first triangle , we will apply "Cosine formula ":

Hence, the shortest distance between the tip of the cone and its rim is 51.15 cm.