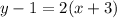Question

Which equation has a graph that is parallel to the graph of 2x - y = -11. 2x + y = 82. y = -1/2x +33. Y - 1 = 2(x -3)4. y = -2x -1

Answer 1

An equation to the line is parallel to other equations if they have the same slope. This slope can be determine if the equation is in the slope-intercept form.

`\begin{gathered} y=mx+b \\ \text{where} \\ m\text{ is the slope} \\ b\text{ is the y-intercept} \end{gathered}`

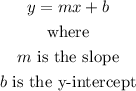
Given the equation 2x - y = -1, convert this equation to slope intercept form.

`\begin{gathered} 2x-y=-1 \\ -y=-2x-1 \\ \text{Divide all terms by negative and we get} \\ y=2x+1 \\ \text{The slope }m\text{ is equal to 2, therefore, we need to find equations whose slope is also} \\ \text{equal to 2} \end{gathered}`

2x + y = 8

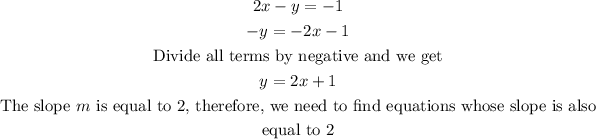
`\begin{gathered} \text{Convert to slope intercept form} \\ 2x+y=8 \\ y=-2x+8 \\ \text{slope is }-2 \end{gathered}`

y = -1/2x + 3, this is already in the slope intercept form where the slope is -1/2.

y - 1 = 2(x-3)

`\begin{gathered} y-1=2(x-3) \\ y=2x-6+1 \\ y=2x-5 \\ \text{the slope is }2 \end{gathered}`

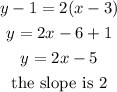
y = -2x - 1, is already in the slope-intercept form with a slope of -2

Conclusion

Out of 4 equations, the equation with the same slope as the given which is 2, is the equation

`y-1=2(x+3)`