Question

a ball is thrown from an initial 5 feet with an initial upward 29 ft per second in the balls height H in feet after T seconds is given by the followingh=5+29t-16t^2find all the values of t for which the balls height is 17 ft round all your answers tk the nearest hundredth.

Answer 1

t=0.64s and t=1.17s

Step-by-step explanation:

The function that models the height of the ball is given below:

`h(t)=5+29t-16t^2`

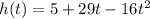
When the ball's height is 17 feet, we have:

`\begin{gathered} 17=5+29t-16t^2 \\ 0=-17+5+29t-16t^2 \\ -16t^2+29t-12=0 \end{gathered}`

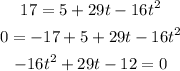
We solve the quadratic equation derived above for the values of t.

We use the quadratic formula.

`x=(-b\pm√(b^2-4ac) )/(2a)`

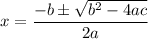
In our own equation: a=-16, b=29, c=-12

`\begin{gathered} t=\frac{-29\pm\sqrt[]{29^2-4(-16)(-12)}}{2(-16)} \\ =\frac{-29\pm\sqrt[]{841-768}}{-32} \\ =\frac{-29\pm\sqrt[]{73}}{-32} \end{gathered}`

Therefore, we have:

`\begin{gathered} t=\frac{-29+\sqrt[]{73}}{-32}\text{ or }t=\frac{-29-\sqrt[]{73}}{-32} \\ t=0.6392\text{ or t=1}.1733 \\ t=0.64\text{ or t=1}.17\text{ (to the nearest hundredth)} \end{gathered}`

The values of t for which the ball's height is 17 ft are 0.64 seconds and 1.17 seconds.