Question

A quantity with an initial value of 600 decays exponentially at a rate of

0.05% every 6 years. What is the value of the quantity after 87 months,
to the nearest hundredth?
Help me

Answer 1

The value of the quantity after 87 months will be of 599.64.

Explanation:

A quantity with an initial value of 600 decays exponentially at a rate of 0.05% every 6 years.

This means that the quantity, after t periods of 6 years, is given by:

`Q(t) = 600(1 - 0.0005)^(t)`

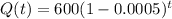
What is the value of the quantity after 87 months, to the nearest hundredth?

6 years = 6*12 = 72 months

So 87 months is 87/72 = 1.2083 periods of 6 years. So we have to find Q(1.2083).

`Q(t) = 600(1 - 0.0005)^(t)`

`Q(1.2083) = 600(1 - 0.0005)^(1.2083) = 599.64`

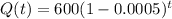
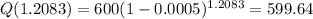
The value of the quantity after 87 months will be of 599.64.