Question

For the following equation determine the value of the missing entries reduce all fractions to lowest term note each column in the table represents an ordered pair. if multiple solutions exist you only need to identify one

Answer 1

Given:

`8x-4y=18`

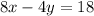
To complete the table, let's substitute the values that are known and find the unknown.

a) y = 0

`\begin{gathered} 8x-4*0=18 \\ 8x=18 \\ Dividing\text{ }both\text{ sides }by\text{ 8:} \\ (8x)/(8)=(18)/(8) \\ x=(18)/(8) \\ Dividing\text{ }the\text{ }numerator\text{ and }the\text{ }denominator\text{ }by\text{ 2:} \\ x=((18)/(2))/((8)/(2)) \\ x=(9)/(4) \end{gathered}`

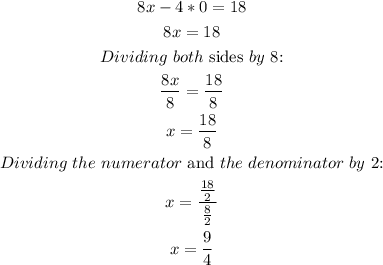
The first point is (9/4, 0).

b) x = 0

`\begin{gathered} 8*0-4y=18 \\ -4y=18 \\ Dividing\text{ }the\text{ }sides\text{ }by\text{ -4:} \\ -(4y)/(-4)=(18)/(-4) \\ y=-(18)/(4) \\ Dividing\text{ }by\text{ }2: \\ y=-((18)/(2))/((4)/(2)) \\ y=-(9)/(2) \end{gathered}`

The first point is (0, -9/2).

c) x = 1

`\begin{gathered} 8*1-4y=18 \\ 8-4y=18 \\ Subtracting\text{ }8\text{ }from\text{ both }sides: \\ 8-4y-8=18-8 \\ -4y=10 \\ Dividing\text{ }the\text{ }sides\text{ }by\text{ }-4: \\ (-4y)/(-4)=(10)/(-4) \\ y=-(10)/(4) \\ Dividing\text{ }the\text{ }sides\text{ }by\text{ 2:} \\ y=-((10)/(2))/((4)/(2)) \\ y=-(5)/(2) \end{gathered}`

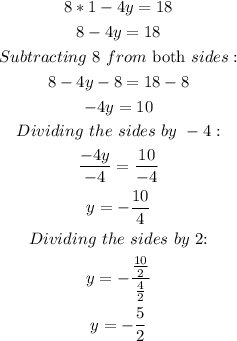
The third point is (1, -5/2).

d) y = 3

`\begin{gathered} 8x-4*3=18 \\ 8x-12=18 \\ Adding\text{ }12\text{ }to\text{ }both\text{ }sides: \\ 8x-12+12=18+12 \\ 8x=30 \\ Divind\text{ }by\text{ 8:} \\ (8x)/(8)=(30)/(8) \\ x=(30)/(8) \\ Divind\text{ }by\text{ 2: } \\ x=((30)/(2))/((8)/(2)) \\ x=(15)/(4) \end{gathered}`

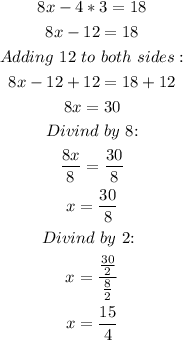
The fourth point is (15/4, 3).

Answer:

x 9/4 0 1 15/4

y 0 -9/2 -5/2 3