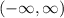Question

PLEASE HELP

A. Identify the parent function of the given graph.
B. In two or more complete sentences, compare and contrast the domain and range of the parent function with the that of the given graph.

Answer 1

The parent function of given graph is
`y=x^3`

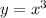
Domain is
`(-\infty,\infty)`

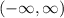
Range is
`(-\infty,\infty)`

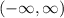
Explanation:

Given the graph below, we have to identify the parent function of given graph.

As the graph of cubic function goes from
`(-\infty)` to
`(\infty)` and also crosses the x-axis minimum 1 time and maximum 3 times. The graph which is given shows the same behavior explained.

∴ the parent function of given graph is
`y=x^3`

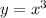
The domain of a function is the complete set of possible values of the independent variable. hence, domain of
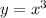`y=x^3` is

The above function is defined at all the values of x

∴ Domain is
`(-\infty,\infty)`

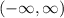
The range of a function is the complete set of all the possible resulting values i.e all y-values.

also Range is
`(-\infty,\infty)`

Answer 2

the parent function of this graph is f(x) = x³. the domain and range of the parent function is all real numbers for both aka (-∞, ∞) in interval notation. there isn't a number you could plug in for x that would give you back an error for this equation.

look at the graph. the range is clearly from (-∞, ∞), as the ends of the function extend in both directions--positive and negative y values--infinitely. as for the domain, it's still (-∞, ∞) or all real numbers. there don't appear to be any cut-offs or limitations with the x-values either. the domain and range of the of the parent function is the same as the function shown on the graph.