Question

Which of the following describes graphing y ≥ |x| + 4?

Translate y = |x| down 4 units and shade inside the V.
Translate y = |x| up 4 units and shade inside the V.
Translate y = |x| left 4 units and shade inside the V.
Translate y = |x| right 4 units and shade inside the V.

Answer 1

Option 2 - Translate y = |x| up 4 units and shade inside the V.

Explanation:

Given : Function
`y\geq |x|+4`

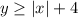
To find : Which of the following describes graphing ?

Solution :

The parent function is
`y=|x|`

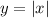
Shift the function 'b' unit up f(x)→f(x)+b

We have given function is increases by 4 unit.

Which means it translated 4 units up.

As y is greater than equal to so it shade inside the V.

Refer the attached figure below.

Translate y = |x| up 4 units and shade inside the V.

Therefore, Option 2 is correct.

Answer 2

Translate
`y=\left|x\right|` up
`4` units and shade inside the V

Explanation:

we know that

The function
`y=\left|x\right|` has the vertex at point
`(0,0)`

The function
`y=\left|x\right|+4` has the vertex at point
`(0,4)`

so

the rule of the translation is

`(x,y)------> (x,y+4)`

That means

The translation is
`4` units up

The solution of the inequality
`y\geq\left|x\right|+4`

is the shaded area inside the V

see the attached figure to better understand the problem

therefore

the answer is

Translate
`y=\left|x\right|` up
`4` units and shade inside the V