Question

What angle measures can the regular octagon be rotated so it maps onto itself?

Select each correct answer.

45°

60°

90°

135°

180°

240°

Answer 1

45°, 90°, 135°, 180°

Explanation:

Answer 2

When a regular octagon is rotated an angle of 45°, 90°, 135°, 180°, it maps onto itself.

Explanation:

When a regular octagon is rotated an angle equal to the measurement of its exterior angle, then the regular octagon maps onto itself.

We know that, the measurement of each exterior angle of a regular polygon of n side is,

`=(360)/(n)`

Here, as the polygon is an octagon, putting n=8,

`=(360)/(8)\\\\=45^(\circ)`

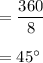
Therefore, when a regular octagon is rotated an angle of 45° or any multiple of 45° i.e 90°, 135°, 180°, it maps onto itself.