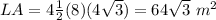Question

A square pyramid has sides of 8 m. Each face is an equilateral triangle. What is the lateral area of the pyramid?

Answer 1

The lateral area is equal to

`LA=64√(3)\ m^(2)`

Explanation:

In this problem the lateral area is equal to the area of one equilateral triangle multiplied by
`4`

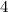
To find the area of one equilateral triangle calculate the height

The area of the triangle is equal to

`A=(1)/(2)bh`

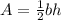
we have

`b=8\ m`

Applying the Pythagoras theorem

`h^(2)=8^(2) -4^(2) \\h^(2)=64-16\\ h=√(48) \\ h=4√(3)\ m`

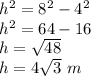
The area of one triangle is equal to

`A=(1)/(2)(8)(4√(3))\ m^(2)`

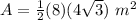
so

The lateral area is equal to

`LA=4(1)/(2)(8)(4√(3))=64√(3)\ m^(2)`