Question

A 2 kg ball is thrown horazontally at 5 m/s from a height of 5 m how fast will it be moving when it touches the ground

Answer 1

The speed of the ball just before it hits the ground is given by:

`v=\sqrt[]{v^2_x+v^2_y}`

the horizontal component of the velocity is constant in the complete trajectory, then:

vx = 5 m/s

the vertical component of the velocity is calculated by using the following formula, for the speed in a free fall motion:

`v^2_y=v^2_(oy)+2gy^{}`

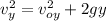
voy is the initial vertical component of the velocity and in this case is

voy = 0m/s.

g: gravitational acceleration constant = 9.8m/s^2

y: vertical distance traveled by the ball = 5 m

Solve the previous equation for vy and replace the values of the other parameters:

`\begin{gathered} v_y=\sqrt[]{2gy} \\ v_y=\sqrt[]{2(9.8(m)/(s^2))(5m)} \\ v_y\approx9.90(m)/(s) \end{gathered}`

Then, by replacing the values of vx and vy, you obtain for v:

`\begin{gathered} v=\sqrt[]{(5(m)/(s))^2+(9.90(m)/(s))^2} \\ v\approx11.10(m)/(s) \end{gathered}`

Hence, the speed of the ball before it hits the ground is approximately 11.10m/s