The given expression is;
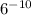
A)

Since bases of both the terms are same i.e., 6
So, when multiplying two powers that have the same base, you can add the exponents.
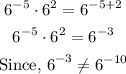
So, Option A is not the right answer;
B)
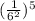
Apply the power rule of exponents;

Thus, Option B is the right answer
C)

Apply the exponents rule;
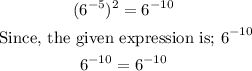
Therefore, Option C is the right answer
D)

Simplify the expression;
since the bases are same so the in the multiplication the exponent value will be add up and during division, the exponent value will be subtract;
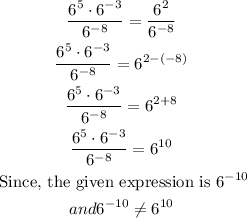
Therefore, Option D is not the right answer
E)

Simplify the expression by using division rule of exponent;
To divide exponents (or powers) with the same base, subtract the exponents:
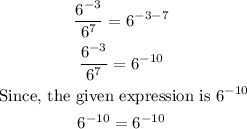
So, option E is the correct answer
Answer : B, C,