Question

The Chandlers are moving across the countryMr. Chandler leaves 5 hours before Mrs. Chandlerhe averages 40 mph and she averages 80 mphhow many hours will it take Mrs. Chandler to catch up to Mr. Chandler?

Answer 1

From the information provided, we know that Mr Chandler travels at a speed of 40 miles per hour (40mph). He also left 5 hours before Mrs Chandler.

Therefore, we can tell that for 5 hours he has travelled

`\begin{gathered} Dis\tan ce=speed\cdot time \\ \text{Distance}=40*5 \\ \text{Distance}=200 \end{gathered}`

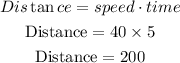
We shall now label the total distance covered as d, and the number of hours taken (time) as t.

We now have for Mr Chandler;

`d=40t+200---(1)`

For Mrs Chandler, we would have;

`d=80t---(2)`

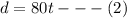
We would now solve the system of equations as follows;

`\begin{gathered} d=40t+200---(1) \\ d=80t---(2) \\ \text{Substitute for the value of d into equation (1)} \\ We\text{ now have;} \\ 80t=40t+200 \\ \text{Collect all like terms;} \\ 80t-40t=200 \\ 40t=200 \\ \text{Divide both sides by 40} \\ t=5 \end{gathered}`

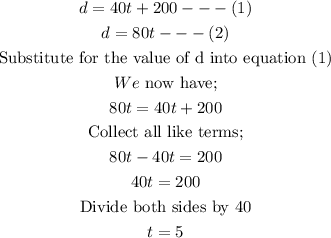
This result shows that, travelling at the speed given for both Mr and Mrs Chandler, it would take her 5 hours to catch up with him.

ANSWER:

5 hours

PART B

If Mr Reeds leaves 3.5 hours ahead of Mrs Reeds and he travels at the speed of 55 miles per hour (55 mph), then his distance would be;

`\begin{gathered} \text{Distance}=\text{spee}d*\text{time} \\ \text{Distance}=55*3.5 \\ \text{Distance}=192.5 \end{gathered}`

If the total distance covered is given as d, then for Mr Reeds the total distance would be;

`d=55t+192.5---(1)`

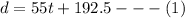
For Mrs Reeds, we would have;

`d=90t---(2)`

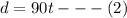
Note that d represents the total distance covered and t represents the time taken (in hours).

We shall now solve the system of equations, as follows;

`\begin{gathered} d=55t+192.5---(1) \\ d=90t---(2) \end{gathered}`

We shall substitute for the value of d into equation two. Note that d = d, which means equation (1) equals equation (2). We now have;

`\begin{gathered} 55t+192.5=90t \\ \text{Collect all like terms;} \\ 192.5=90t-55t \\ 192.5=35t \\ \text{Divide both sides by 35} \\ (192.5)/(35)=(35t)/(35) \\ 5.5=t \end{gathered}`

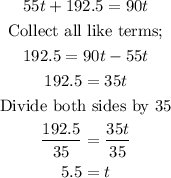
The calculations here shows that travelling at the speeds given for both of them, it would take Mrs Reeds 5.5 hours to catch up with Mr Reeds.

ANSWER:

5.5 hours