Question

Select the statement that accurately describes the following pair of triangles 1. ABC~YZX~by SAS2. ABC~YXZ~by SAS3. ABC~ZYX~by SAS4. ABC~ZXY~by SAS5.ABC~XZY~by SAS6. ABC~XYZ~by SAS7. Triangles are not similar

Answer 1

Step 1: Let's recall what is SAS:

"SAS" means "Side, Angle, Side"

Step 2: Let's use The Law of Cosines to calculate the unknown side of the triangles given:

Formula of The Law of Cosines is:

`a^2=b^2+c^2\text{ - }2\text{ bc }\cdot\text{ }\cos \text{ (}\angle A\text{)}`

In the triangle ABC, we have:

b = 15, c = 21 and angle A is 75 degrees

Step 3: Substitute the values given in the formula

`a^2=15^2+21^2\text{ - 2 }\cdot\text{ 15 }\cdot\text{ 21 }\cdot\mathring{Cos(75}\circ)`
`a^2\text{ = 225 + 441 - }630\ast\text{ 0.2588}`
`a^2\text{ = 666 - 163.044 = 502.956 }\Rightarrow\text{ a = 22.43}`

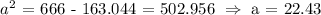
Step 4: Let's use The Law of Sines to find the smaller of the other two angles

`\sin \text{ }\angle B\text{ }(\square)/(\square)21\text{ }=\text{ }\sin \text{ (75) }(\square)/(\square)\text{ 22.43}`
`\sin \text{ }\angle B=\text{ (}0.966\text{ }\ast\text{ 21) / 22.43}`
`\sin \angle\text{ B = 0.9044 }\Rightarrow\text{ B = }\sin ^(-1)\text{ (0.9044)}`
`\angle B\text{ = 64.74}\circ\text{ }\Rightarrow\text{ }\angle C\text{ = 180 - 64.74 - 75 = 40.26}\circ`

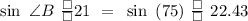
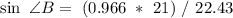
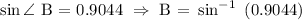
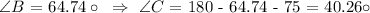
Step 5: Find the last angle, recalling that the interior angles of a triangle add up to 180 degrees

`\angle\text{ C = 180 - 64.74 - 75 = 40.26}\circ`

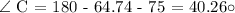
Now, we have the sides and the angles of triangle ABC:

Sides = 15,21, 22.43

Angles = 75, 64.74, 40.26