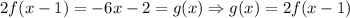To answer this question, we have that both functions are linear functions defined in some intervals. We can find the line equations for those lines as follows:
Finding function f in the interval [-2, -1]
1. We need to define the function f using the points:
(-2, 2) and (-1, -1).
Using these points, we can find the line equation using the two-point form of the line equation:
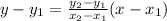
(-2, 2) ---> x1 = -2, y1 = 2
(-1, -1) ---> x2 = -1, y2 = -1
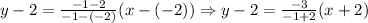
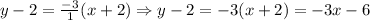
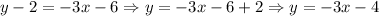

Therefore, the function f(x) = -3x - 4 in the interval [-2, -1]
Finding the function g in the interval [-1, 0]
To find the function g, we can proceed in a similar way:
1. We have the following points:
(-1, 4) and (0, -2)
Then, we have:
(-1, 4) ---> x1 = -1, y1 = 4
(0, -2) ---> x2 = 0, y2 = -2
2. Applying the two-point form of the line, we have:
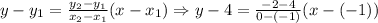
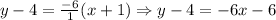
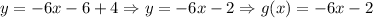
Therefore, the function g(x) = -6x - 2 in the interval [-1, 0].
If we use the given options in the question, we have that:
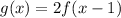
We have that:

Then, we have:

Then
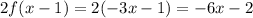
Therefore