Question

A Sno-Cone at the carnival has the shape of a hemisphere on top of an inverted cone. What is the volume ofthe sno-cone if its radius is 5.5 mm and the height of the conical portion is 9.7 mm?

Answer 1

Volume = 655.73

Step-by-step explanation:

The sno-cone is composed of a hemisphere and a cone; therefore, its volume is

`V=V_(cone)+V_(hemisphere)`

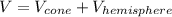
Now

`V_{\text{cone}}=(\pi r^2h)/(3)`

and the volume of the hemisphere is

`V_{\text{hemisphere}}=(2)/(3)\pi r^3`

Therefore,

`V=V_(cone)+V_(hemisphere)`
`\rightarrow V=(\pi r^2h)/(3)+(2)/(3)\pi r^3`

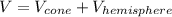
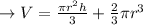
putting in h = 9.7 mm and r = 5.5 mm gives

`\begin{gathered} V=(\pi(5.5)^2(9.7))/(3)+(2)/(3)\pi(5.5)^3 \\ \end{gathered}`

which simplifies to give (rounded to the nearest hundreth)

which is our answer!