Question

For the following true conditional statement, write the converse. If the converse is also true, combine the statements as a biconditional.

If x = 9, then x2 = 81.

a)If x2 = 81, then x = 9.
b)If x2 = 81, then x = 9.
x2 = 81 if and only if x = 9.
c)If x2 = 9, then x = 81.
d)If x2 = 81, then x = 9.
x = 9 if and only if x2 = 81

Answer 1

x2 = 81 if and only if x = 9.
this statement means

x2 = 81 implies x = 9, and x = 9 implies x²=81
so the converse of If x = 9, then x2 = 81 is If x2 = 81, then x = 9

Answer 2

The converse statement interchange the hypothesis and the conclusion.

The converse of x=9 then
`x^(2) =81.` is
`x^(2) = 81 then x=9.` is true.

A biconditional statement is defined to be true whenever both parts have the same truth value . combining the statements as a biconditional we have

If
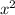`x^(2)`. = 81, then x = 9.

x = 9 if and only if
`x^(2) .` = 81

Option D is the right answer.