Question

How does the graph of g(x) 1/x+4-6 compare to the graph of the parent function f(x) 1/x

g(x) is shifted 4 units right and 6 units up from f(x).
g(x) is shifted 4 units right and 6 units down from f(x).
g(x) is shifted 4 units left and 6 units up from f(x).
g(x) is shifted 4 units left and 6 units down from f(x).

Answer 1

g(x) is shifted 4 units left and 6 units down from f(x).

Answer 2

Option 4- Graph of g(x) is shifted 4 units left and 6 units down from f(x).

Explanation:

Given : Graph of
`g(x)=(1)/(x+4)-6`

To find : How does the graph of g(x) compare to the graph of the parent function
`f(x)=(1)/(x)`

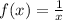
Solution :

The graph f(x) is being translated to form the graph g(x).

In graph
`g(x)=(1)/(x+4)-6` there is

Translation of 4 unit left and 6 unit downward.

In graph f(x) if we shift the graph with 4 unit left it form

f(x)→f(x+b) , the function is shifted towards left with b unit.

So,
`f(x)=(1)/(x+4)`

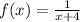
In graph f(x) if we shift the graph with 6 unit downward it form

f(x)→f(x)-a , the function is shifted downward with a unit.

So,
`f(x)=(1)/(x+4)-6`

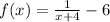
Therefore, Option 4 is correct.

Graph of g(x) is shifted 4 units left and 6 units down from f(x).