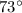Question

What is the measure of angle ACB?

Answer 1

Linear pair is a pair of adjacent angles formed when two lines intersect.

so,
`\angle EAB` and
`\angle CAB` are linear pairs.

Linear pairs are always supplementary.

therefore,

`\angle EAB+\angle CAB=180^(\circ)` ......[1]

Also, it is given in figure,
`\angle EAB = 108^(\circ)`

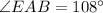
Substitute in [1], we get;

`108^(\circ)+\angle CAB=180^(\circ)`

Simplify:

`\angle CAB =180^(\circ)-108^(\circ)=72^(\circ)`

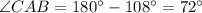
Exterior- Angle sum Property states that if the measure of an exterior angle of a triangle is equal to the sum of the measures of the two non-adjacent interior angles of the triangle.

then by Exterior angle sum property ;

`\angle ACB+\angle CAB =\angle CBF`

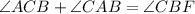
Substitute the values given in the figure;

`\angle ACB+72^(\circ)=145^(\circ)`

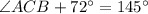
simplify;

`\angle ACB =145-72 = 73^(\circ)`

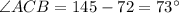
therefore, the measure of angle ACB is,
`73^(\circ)`

Answer 2

The answer is 73 degrees.... A triangle adds up to 180 degrees. The angle next to B is a supplementary angle so you subtract 180 - 145= 35.... angle next to A is also supplementary so you 180 - 108 = 72

72 + 35 = 107
180 - 107= 73

Your answer is 73 degrees