Question

A right triangle is removed from a rectangle to create the shaded region shown below. Find the area of the shaded region. Be sure to include the correct unit in your answer.

Answer 1

The area of shaded region is 45 square units.

Explanation:

The length of the rectangle is 8 and the breadth is 6.

Area of rectangle is

`A_R=l* b`

`A_R=8* 6=48`

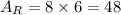
The area of rectangle is 48 square units.

Since the opposite sides of a rectangle are equal, therefore the legs of the right angled triangle are

`l_1=8-5=3`

`l_2=6-4=2`

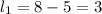
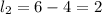
The area of right angled triangle is

`A_T=(1)/(2)* l_1* l_2`

`A_T=(1)/(2)* 3* 2=3`

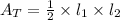
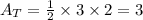
The area of triangle is 3 square units.

The area of shaded region is

`A=A_R-A_L=48-3=45`

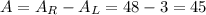
Therefore the area of shaded region is 45 square units.

Answer 2

The sides of a right triangle are

`a=8-5=3 \\ b=6-4=2 \\ \\A=A_(rectangle)-A_(triangle)=8* 6- (ab)/(2) =48-(3*2)/(2)=48-3=45`