Question

Bradley and Kelly are out flying kites at a park one afternoon. A model of Bradley and Kelly's kites are shown below on the coordinate plane as kites BRAD and KELY, respectively:

Which statement is correct about the two kites?

They are similar because Segment BR to segment DB is 1:2 and Segment KE to segment YK 1:2.

They are not similar because Segment BR to segment DB is 1:5 and Segment KE to segment YK is 1:2.

They are not similar because Segment BR to segment DB is 1:2 and Segment KE to segment YK is 1:5.

They are similar because Segment BR to segment DB is 1:5 and Segment KE to segment YK is 1:5.

Answer 1

3rd answer. They are not similar because BR-DB has a larger ratio then KE-YK. Similar means the corresponding sides have the same ratios between 2 shapes. The sides do not.

Answer 2

1. The vertices B, D and R have coordinates (9,9), (1,5) and (11,5), respectively. Then

`BR=√((11-9)^2+(5-9)^2)=√(4+16) = 2√(5) ,\\BD=√((1-9)^2+(5-9)^2)=√(64+16) = 4√(5).`

The ratio

`(BR)/(BD)= (2√(5))/(4√(5)) =(1)/(2).`

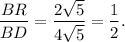
2. The vertices K, E and Y have coordinates (13,9), (14,10) and (14,2), respectively. Then

`KE=√((13-14)^2+(9-10)^2)=√(1+1) = √(2) ,\\KY=√((13-14)^2+(9-2)^2)=√(1+49) = 5√(2).`

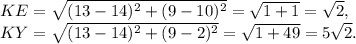
The ratio

`(KE)/(KY)= (√(2))/(5√(2)) =(1)/(5).`

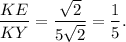
Answer: correct answer is C (they are not similar because segment BR to segment DB is 1:2 and segment KE to segment YK is 1:5.)