Question

How long will it take for an investment of 1600 dollars to grow to 7600 dollars, if the nominal rate of interest is 8.3 percent compounded quarterly? FV = PV(1 + r/n )^ntAnswer= ________years. (Be sure to give 4 decimal places of accuracy.)

Answer 1

18.9669 years

Step-by-step explanation:

principal = $1600

future value = $7600

rate = 8.3% = 0.083

n = number of times compounded = quarterly

n = 4

time = ?

To determine the time it will take, we will apply the compound interest formula:

`FV\text{ = P(1 +}(r)/(n))^(nt)`

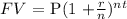
substitute the values into the formula:

`\begin{gathered} 7600\text{ = 1600(1 +}(0.083)/(4))^(4* t) \\ 7600=1600(1+0.02075)^(4t) \\ \\ \text{divide both sides by 1600:} \\ (7600)/(1600)=(1600(1+0.02075)^(4t))/(1600) \\ 4.75\text{ = }(1+0.02075)^(4t) \\ \end{gathered}`
`\begin{gathered} 4.75\text{ = }(1.02075)^(4t) \\ \text{take log of both sides:} \\ \log 4.75\text{ = log }(1.02075)^(4t) \\ \log 4.75\text{ = 4t log }(1.02075) \\ \\ \text{divide both sides by log }(1.02075)\colon \\ \frac{\log 4.75\text{ }}{\text{ log }(1.02075)}\text{=}\frac{\text{ 4t log }(1.02075)}{\text{ log }(1.02075)} \\ 75.8677\text{ = 4t} \end{gathered}`
`\begin{gathered} \text{divide both sides by 4:} \\ (75.8677)/(4)\text{ = }(4t)/(4) \\ t\text{ = 18.9669} \end{gathered}`

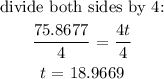
It will take 18.9669 years (4 decimal place)