Question

Show steps on finding x,y int and end behaviors of a polynomial.

Answer 1

To find the y-intercept we have to evaluate the given function at x=0:

`\begin{gathered} f(0)=(0-1)^3(0+3)^2(0-4)(0+2),^{} \\ f(0)=(-1)^3(3^{})^2(-4)(2), \\ f(0)=72. \end{gathered}`

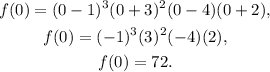
Therefore, the coordinates of the y-intercept are (0,72).

To find the x-intercepts we set f(x)=0 and solve for x:

`\begin{gathered} (x-1)^3(x+3)^2(x-4)(x+2)=0. \\ \Leftrightarrow x=1\text{ or x=-3 or x=4 or x=-2.} \end{gathered}`

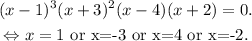
The x-intercepts have coordinates (1,0), (-3,0), (4,0), (-2,0).

Finally, the end behaviours are:

`\begin{gathered} \lim _(x\rightarrow+\infty)f(x)=\lim _(x\rightarrow+\infty)(x-1)^3(x+3)^2(x-4)(x+2)=+\infty, \\ \lim _(x\rightarrow-\infty)f(x)=\lim _(x\rightarrow-\infty)(x-1)^3(x+3)^2(x-4)(x+2)=-\infty. \end{gathered}`

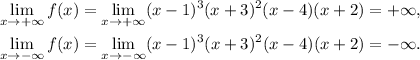
Answer: