Question

Factor the polynomial F(x) = x^3 − x^2 − 4x + 4 completely. Lesson 5.4.1Part I: Find and list all the possible roots of F(x) (pq) Show your work. Part II: Use the Remainder Theorem to determine at least one of the roots of F(x) from Part I. Show your work.Part III: Find the remaining factors F(x) = x3 − x2 − 4x + 4 completely. Show your work.

Answer 1

Given

`x^3−x^2−4x+4`

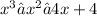
Step-by-step explanation

Part 1: Since the constant in the given equation is 4,the integer root must be a factor of 4. The possible values are

Answer:

`\pm1,\pm2,\pm4`

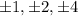
Part 2: The remainder theorem says when a polynomial a(x) is divided by a linear polynomial b(x) whose zero is x = k, the remainder is given by r = a(k). In this case for one of the possible values to be a root, it must give a zero result when inserted in the original expression.

Therefore,

`\begin{gathered} when\text{ }x=1 \\ f(1)=1^3-(1)^2-4(1)+4=1-1-4+4=0 \\ when\text{ }x=2 \\ f(2)=2^3-(2)^2-4(2)+8=8-4-8+4=0 \end{gathered}`

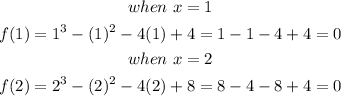
Answer: Therefore, 1 and 2 are roots of the polynomial

Part 3:

Let the last factor of the polynomial be ax+b

Therefore,

`\begin{gathered} (x-1)(x-2)(ax+b)=x^3-x^2-4x+4 \\ By\text{ comparison of terms} \\ ax^3=x^3 \\ \therefore a=1 \\ Also \\ 2b=4 \\ b=(4)/(2)=2 \end{gathered}`

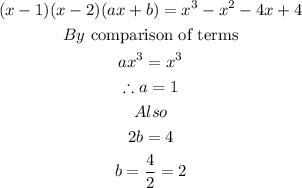
The factors of the equation are therefore;

Answer: (x-1)(x-2)(x+2)