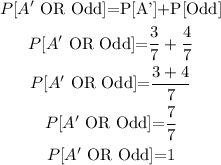From the information provided, the diagram would appear as follows;
The sample space of the spinner i;

(2) What is set A?
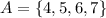
(3) What is the probability of spinning a number greater than or equal to 4.
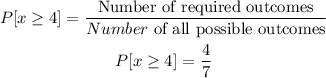
(4) What is set B?
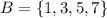
(6) What is A and B?
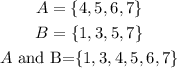
(5) What is the probability of spinning an odd number?
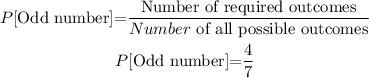
(7) What is the probability of spinning a number greater than or equal to 4 and odd?

(8) What is the probability of spinning a number NOT greater than or equal to 4
or an odd number?
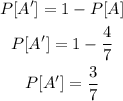

Therefore;