Question

What is the length of the altitude of the equilateral triangle below

Answer 1

F. 12

Explanation:

We have been given an image of a triangle and we are asked to find the length of the altitude of our given triangle.

Since we know that altitude of an equilateral triangle splits it into two 30-60-90 triangle.

We will use Pythagoras theorem to solve for the altitude of our given triangle.

`\text{Leg}^2+\text{Leg}^2=\text{Hypotenuse}^2`

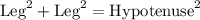
Upon substituting our given values in above formula we will get,

`(4√(3))^2+a^2=(8√(3))^2`

`16*3+a^2=64*3`

`48+a^2=192`

`48-48+a^2=192-48`

`a^2=144`

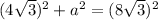
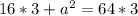
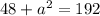
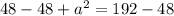
Upon taking square root of both sides we will get,

`a=√(144)`

`a=12`

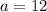
Therefore, the length of the altitude of our given equilateral triangle is 12 units and option F is the correct choice.

Answer 2

Find out the altitude of the equilateral triangle .

To proof

By using the trignometric identity.

`tan\theta = (Perpendicular)/(base)`

As shown in the diagram

and putting the values of the angles , base and perpendicular

`tan 60^(\circ) = (a)/(4√(3))`

`tan 60^(\circ) = √(3)`

solving

`√(3) = (a)/(4√(3))`

`a = √(3)* 4 √(3)`

As

`√(3)* √(3) = 3`

put in the above

a = 4 × 3

a = 12 units

The length of the altitude of the equilateral triangle is 12 units .

Option (F) is correct .

Hence proved