Question

A can of beans has surface area 312 cm^2. Its height is 11 cm. What is the radius of the circular top?The radius of the circular top is ___cm.(Do not round until the final answer. Then round to the nearest hundredth as needed.)

Answer 1

Answer: The radius of the circular top is 3.45cm

Step-by-step explanation

• Surface area (SA): 312cm².

,

• Height (h): 11cm.

A can of beans can be approximated to a cylinder figure. Then, the cylinder has a formula for its surface area (SA), which is:

`SA=2\pi rh+2\pi r^2`

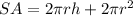
where r represents the radius and h represents the height.

As we have the value for SA and h we can replace them in the formula:

`312=2\pi r(11)+2\pi r^2`

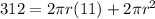
Simplifying the expression by multiplying the parenthesis:

`312=22\pi r+2\pi r^2`

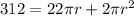
As we have a second-degree polynomial, we can use the General Quadratic Formula to find the solution for r. In order to do so, we have to set the equation to 0 as follows:

`0=ax^2+bx+c`

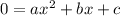
where a, b and c are used in the General Quadratic Formula:

`r_(1,2)=(-b\pm√(b^2-4ac))/(2a)`

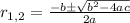
In our case, our equation is:

`0=2\pi r^2+22\pi r-312`

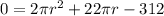
where a = 2π, b = 22π, and c = -312.

Then, replacing these values in the General Quadratic formula we get:

`r_(1,2)=(-22\pi\pm√((22\pi)^2-4(2\pi)(-312)))/(2(2\pi))`

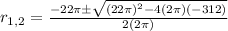
Simplifying the terms inside the square root and the denominator:

`r_(1,2)=(-22\pi\pm√((22\pi)^2-2496\pi))/(4\pi)`
`r_(1,2)=(-22\pi\pm√(12618))/(4\pi)`

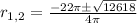
Finally, finding both solutions:

`r_1=(-22\pi+112.33)/(4\pi)\approx3.44`
`r_2=(-22\pi-112.33)/(4\pi)=-14.44`

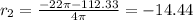
As we cannot have a negative radius, then the correct answer is that the radius measures 3.44cm