Answer:
a)
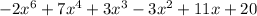
b) The product of (-2x^3 + x - 5 ) and (x^3 - 3x - 4 ) is equal to the product of (x^3 - 3x - 4) and (-2x^3 + x - 5 ) since, according to the commutative property of multiplication, changing the order of the numbers being multiplied does not change the product.
Step-by-step explanation:
Given the expressions;
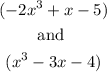
a) To determine the product of the above expressions, we have to use each term in the first expression to multiply each term in the second expression as seen below below;
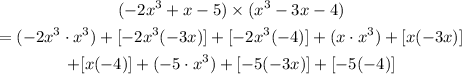
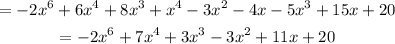
So the product of (-2x^3 + x - 5 ) and (x^3 - 3x - 4 ) is;
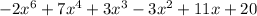
b) Note that the commutative property of multiplication states that changing the order of the numbers being multiplied does not change the product.
So we can say that the product of (-2x^3 + x - 5 ) and (x^3 - 3x - 4 ) is equal to the product of (x^3 - 3x - 4) and (-2x^3 + x - 5 ) since, according to the commutative property of multiplication, changing the order of the numbers being multiplied does not change the product.