Question

Find the derivative of sec3t/tan5t

Answer 1

We will need to use Quotient Rule along with the chain Rule.
Also we need to know the basic derivatives of sec and tan.


`(d)/(dt) sec(u) = sec(u) tan(u) (du)/(dt)`

`(d)/(dt) tan(u) = sec^2 (u) (du)/(dt)`

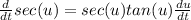
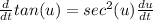
We will refer to those later.
Now lets look at the Quotient rule:

`((f)/(g))' = (f' g - fg')/(g^2)`
f(t) = sec(3t) , g(t) = tan(5t)
Find f'(t) and g'(t) using derivatives from top of post.
Note that u = 3t for f(t) and u = 5t for g(t).

`f'(t) = 3sec(3t) tan(3t)`

`g'(t) = 5 sec^2 (5t)`
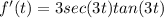
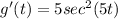
Substituting into quotient formula:

`(3 sec(3t) tan(3t) tan(5t) - 5 sec(3t) sec^2 (5t))/(tan^2 (5t))`