Question

What is the number of real solutions?

–11x2 = x + 11

cannot be determined
one solution
two solutions
no real solutions

Answer 1

no real solutions.

Explanation:

-11
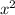`x^(2)` = x+11. Put into standard form of a quadratic equation.

11
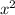`x^(2)` + x + 11 = 0 Find a, b, and c.

a = 11, b =1, c = 11 Write and substitute into the quadratic equation
`\frac{-b+/-\sqrt{b^(2)-4ac}}{2a}` .

`\frac{-1 +/- \sqrt{1^(2) - 4(11)(11)} }{2(11)}` Simplify.

`(-1 +/- √(1-484) )/(2(11))`=

`(-1 +/-√(-483) )/(22)` BUT because the
`b^(2) -4ac` part under the √ is less than 0, THERE IS NO SOLUTION!

Answer 2

I assume the x between -11 and 2 is multiply

that would be -22=x+11
x=-33

so there's only one solution.